
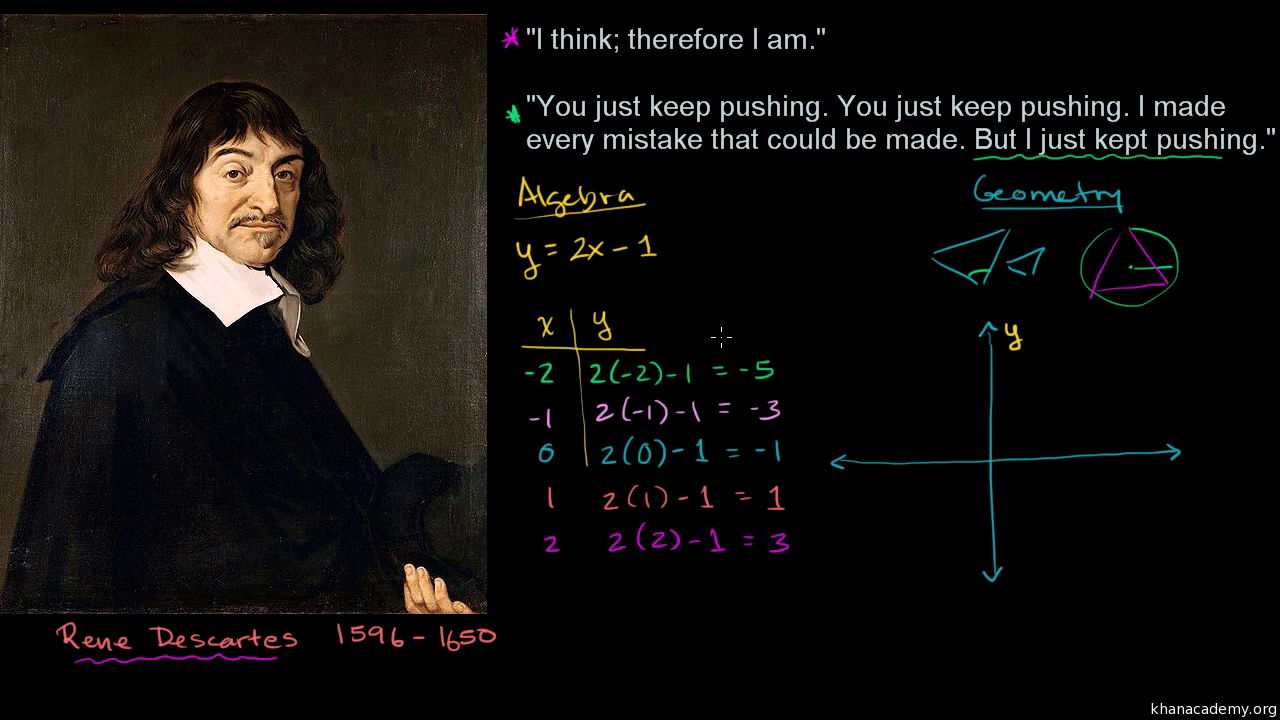
And one way to interpret that, for whatever amount you increase in the horizontal direction, Here, the slope of that line, is going to be equal to two. Is equal to the slope of this magenta line. So six two over one isĮqual to six over three is equal to two, this We measure the increase in vertical for a given Used for a ski slope, and that's because a ski slope And you're probablyįamiliar with the notion of the word slope being

Increase in vertical divided by increase in horizontal, this is what mathematicians In the horizontal direction by a given amount, I'm going to increase twice as much twice as much in the vertical direction. So no matter where I start on this line, no matter where I start on this line, if I take and if I increase Hey, let's just measure how much to we increase in vertical for a given increase in the horizontal? Well two over one is just two and that's the same So when I increase by three in the horizontal direction, I increase by six in the vertical. Two, three, four, five, six I have to increase by six. In the vertical direction? I have to increase by one, So now, I've gone plus three in the horizontal direction, then to get back on the line, how much do I have to increase Increasing the horizontal direction by one, if I were increase in the horizontal direction. Still work if I were to start here, instead of So at least for this magenta line, it looks like our increase in vertical is two, whenever we have an increase in one in the horizontal direction.

The vertical direction? Well I have to increase in To get back on the line, how much do I have to increase in So if we were to start right here, and if I were to increase But I'll start at a point where it's going to be easy for me to figure out what point we're at. Now let's just start at an arbituary point in that magenta line. So, how can this give us a value? Well let's look at that So let's say if we an increase increase, in vertical, in vertical, for a given increase in horizontal for a given increase a given increase in horizontal. The horizontal direction? So let's write this down. Or to any lines that describes how steep it is, how quickly does it increase or decrease? So what's a reasonable way to do that? What's a reasonable way to assign a number to these lines thatĭescribe their steepness? Well one way to thinkĪbout it, could say well, how much does a line increase in the vertical direction for a given increase in
So ideally, we'd beĪble to assign a number to each of these lines Quickly does it increase or how quickly does it decrease, is a really useful idea in mathematics. This notion of steepness, how steep a line is, how This magenta line here, it looks steeper than this blue line. As we start to graph lines, we might notice that they'reĭifferences between lines.


 0 kommentar(er)
0 kommentar(er)
